中緯度大気変動の力学 −中立モードを中心に−

タイトルぺージ

中高緯度大気の長周期変動

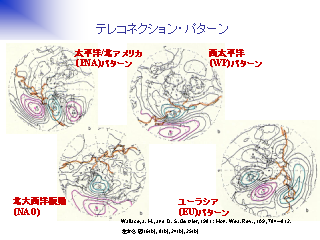
図は Wallace, and Gutzler, 1981

テレコネクションパターン 冬の 12 月月平均 500 hPa 等圧面高度の一点相関図: 図中の赤点で示した地点と他の地点との相関 冬の北半球に特徴的な気圧場のパターンをはじめて示した

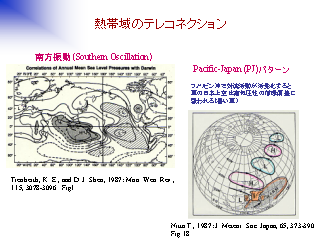
熱帯域のテレコネクション

PNA パターンとエルニーニョ 熱帯の大気強制に対する応答として解釈 エルニーニョの場合の大気場の観測結果に基づく

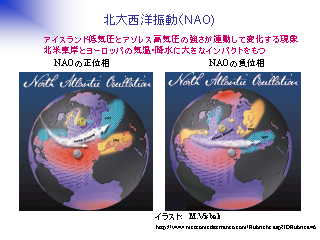
北大西洋振動 (NAO)

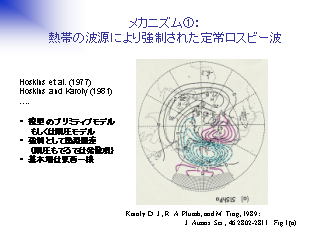
メカニズム1: 熱帯の熱源により強制された定常ロスビー波 線形プリミティブモデルの強制に対する応答として考える 熱源を与えて大気の応答を見る 基本場の風速は観測された東西平均値を与える 定常ロスビー波の伝播の様子はテレコネクションパターンによく似ている

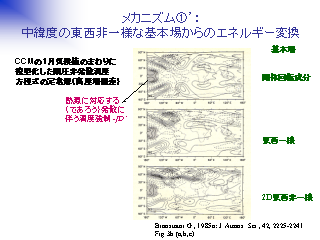
メカニズム1: 中緯度の東西非一様な基本場からのエネルギー変換 基本場の風速が東西一様でない場合: 基本場の風は北半球の 1 月 観測値から抜きだした剛体回転場: 南北対称な応答が現れる 東西一様場: 同じ緯度に熱源を与えると経度によらず同じ応答が現れる (アタリマエ) 東西非一様場: 基本場は東西非一様だが, 同じ緯度に熱源を与えると経度によらず同じ応答 (地理的に同じ位置に同じパターン)が現れる (なぜだろう?) ずらす経度幅の程度による. インド洋から中部太平洋の範囲では同じ.

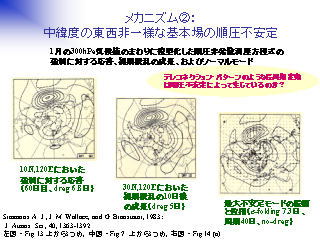
メカニズム2: 中緯度の東西非一様な基本場の順圧不安定 あまりうまくない: 現実的な摩擦を与えた場合, 実効的な成長率が小さい 結果的には中立モードによる理解と同じ見方 ?

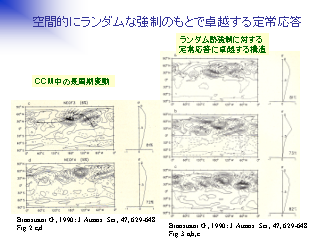
空間的にランダムな強制のもとで卓越する定常応答 (左)大気大循環モデルの結果 (EOF3, EOF4) (右)線形定常プリミティブモデルの結果 300 hPa 等圧面高度 (北半球冬) 空間的にランダムな分布の強制を与え線形応答問題を解く. 200 の異なる初期値に対する結果を平均 強制源の空間パターンに規則性がなくても, 大気の気圧パターンにはある特徴的な構造が応答として現れる.

まとめ・疑問点・問題点など 観測値から得られた特徴的な気圧パターン (EOF) に, なんらかの力学的解釈を与えれないだろうか? EOF は線形大気モデルの固有関数に対応するのか?

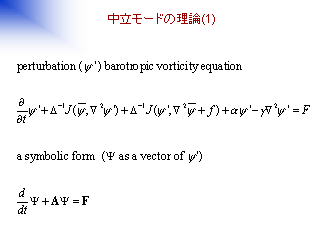
中立モードの理論 Dymnikov (1988) がさきがけ 西側の科学者では Navarra (1993)

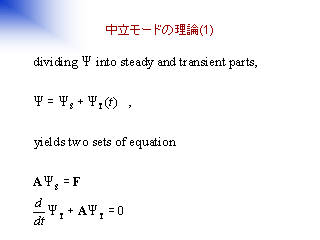
中立モードの理論 1-1 Ψ の平均場は適当な強制によって維持されると考える.

中立モードの理論 1-2 Ψ を強制定常解と斉次解に分ける

中立モードの理論 1-3 Ψ の斉次解部分を固有振動数と固有ベクトルで表現できると仮定

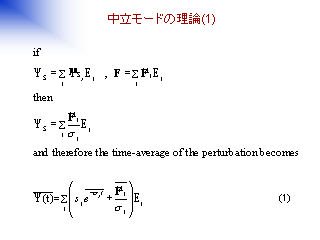
中立モードの理論 1-4 時間平均は適当な有限時間で行う

中立モードの理論 1-5

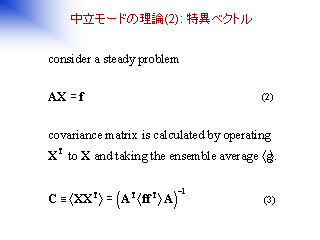
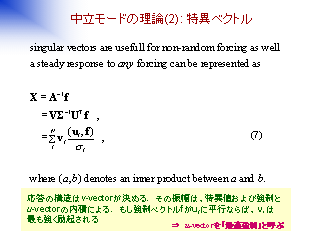
中立モードの理論 2-1: 特異ベクトル 観測データから抽出されたパターン (ex. EOF) をどうとらえるか? 与えられた力学系の係数行列の固有ベクトルが直交しない場合, その他のなんらかの直交ベクトルで理解できないか, を考える.

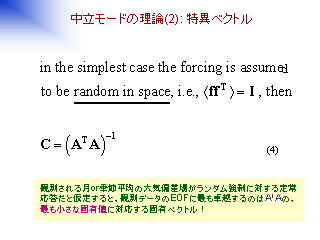
中立モードの理論 2-2 強制がランダムな空間パターンを持つ場合

中立モードの理論 2-3

中立モードの理論 2-4

中立モードの理論 2-5

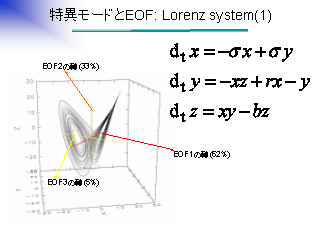
特異モードと EOF: Lorenz system (1)

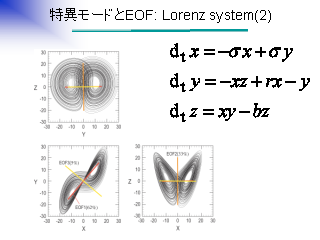
特異モードと EOF: Lorenz system (2)

特異モードと EOF: Lorenz system (3)

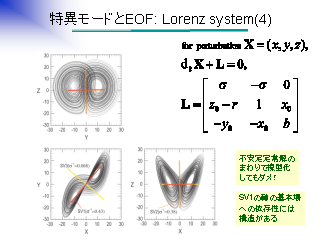
特異モードと EOF: Lorenz system (4)

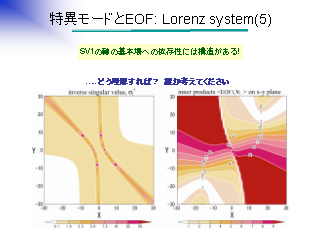
特異モードと EOF: Lorenz system (5)

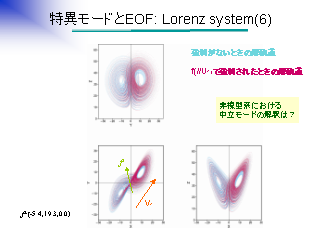
特異モードと EOF: Lorenz system (6)

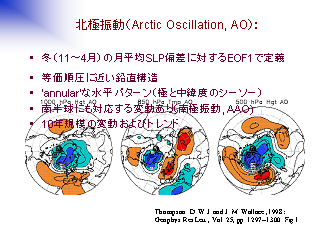
中立モードの計算例その 1: 北極振動

北極振動 (AO) とは ?

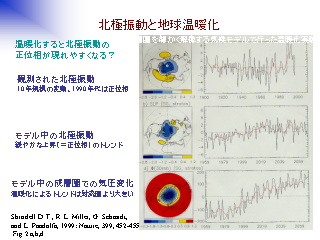
AO のメカニズムについて

北極振動と地球温暖化

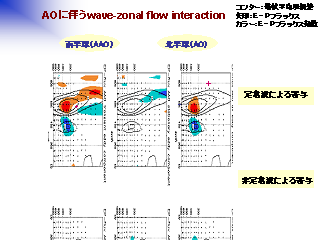
AO にともなう波動平均流相互作用 月より長い周期と短い周期の波による平均東西風の加速寄与 月より長い周期の波の寄与が大きい

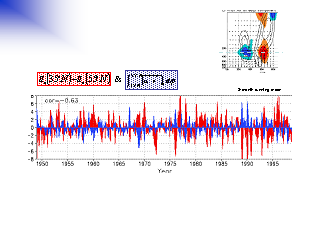
南向き運動量輸送と平均風速偏差の南北差との関係 南向きの運動量輸送 (青) と平均風速偏差の南北差 (赤) によい相関がある.

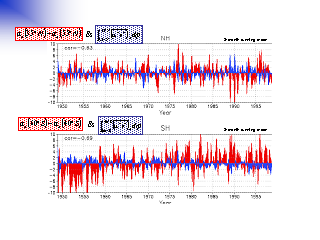
南北の運動量輸送と平均風速偏差の南北差との関係 (上) 北半球 (下) 南半球

AO の力学的起源を調べるための戦略

線形傾圧モデル モデル概要

モデル方程式

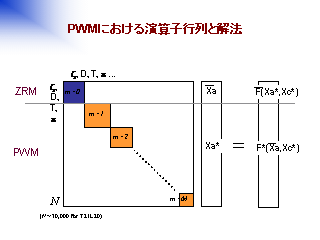
PWM における演算子行列と解法

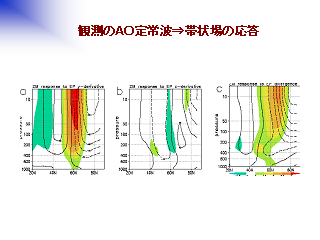
観測の AO 定常波と帯状場の応答 (北半球東西平均場)

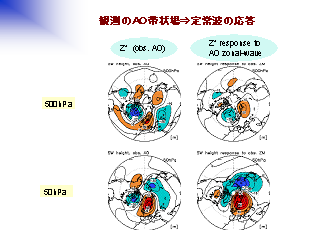
観測の AO 定常波と帯状場の応答 (北半球)

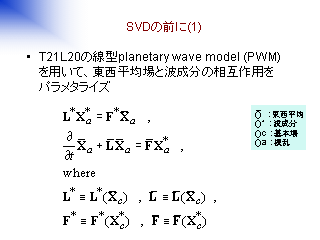
SVD の前に (1)

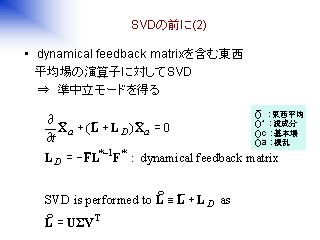
SVD の前に (2)

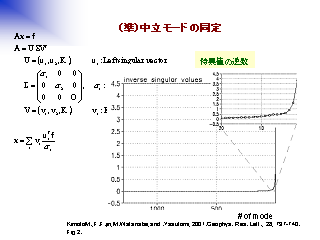
中立モードの同定 一番低次のモードの特異値が最も小さい

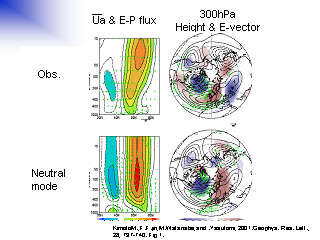
中立モードと観測結果との比較 基本的なパターンはよく似ている

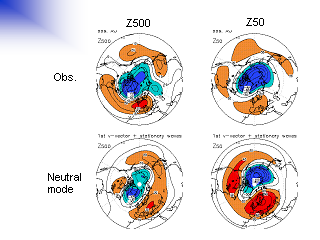
観測される AO と中立モードの水平構造の比較

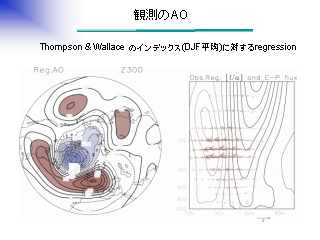
観測される AO

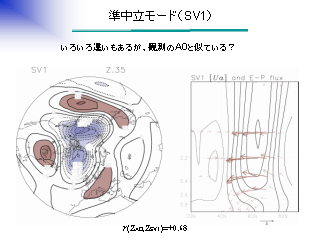
準中立モード 空間構造は観測される AO に似ている?

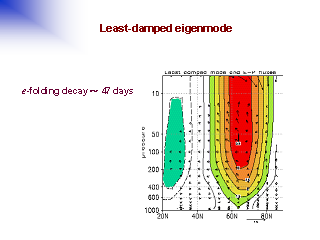
準中立モードの子午面構造

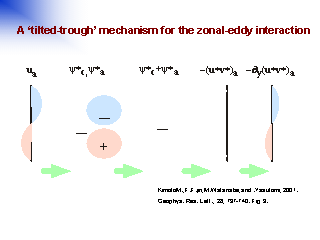
波による加速機構 平均風のシアーによって波の位相が傾き, 平均風シアーを強める

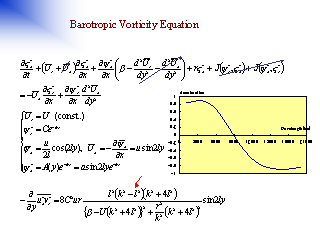
順圧渦度方程式

まとめ 線形のプリミティブモデルにおける特異値分解から, AO に近い波のパターンを得ることができる 力学的には AO は NAO とは独立であると判断できる?

モード計算に含まれなかったもの

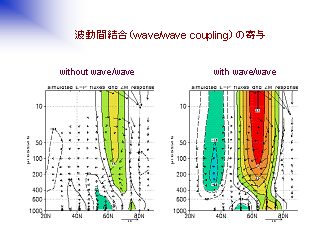
波動間結合の寄与

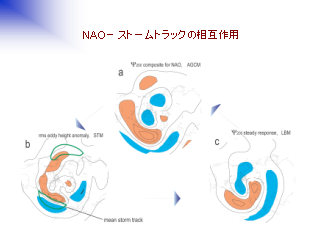
NAO とストームトラックの相互作用 非定常な波の寄与を考慮した場合

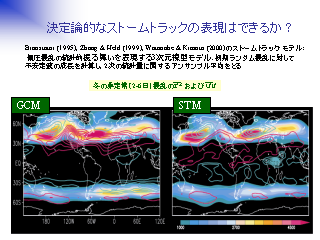
決定論的なストームトラックの表現はできるか?

中立モード計算その 2: 3 次元基本場のもとでのモード

ここまでの結果

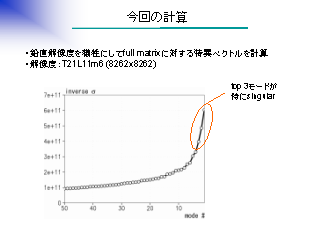
今回の計算

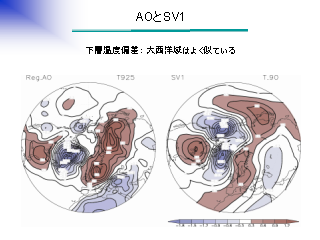
AO と SV1

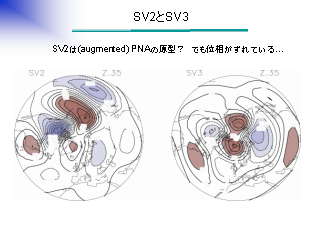
SV2 と SV3

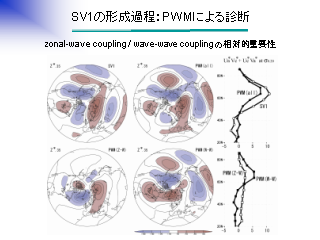
SV1 の形成過程: PWM による診断 (1)

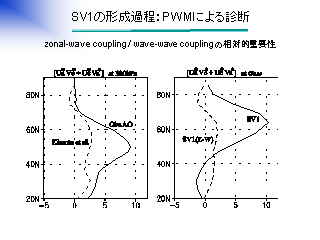
SV1 の形成過程: PWM による診断 (2)

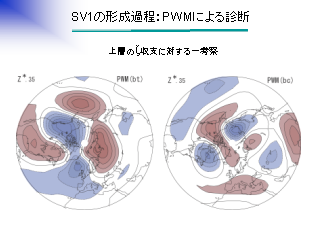
SV1 の形成過程: PWM による診断 (3)

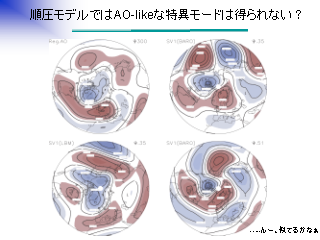
順圧モデルでは AO-like な特異モードは得られない?

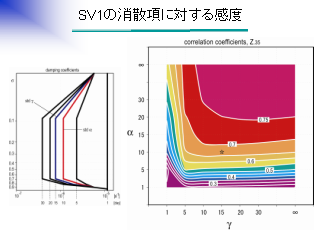
SV1 の消散項に対する感度

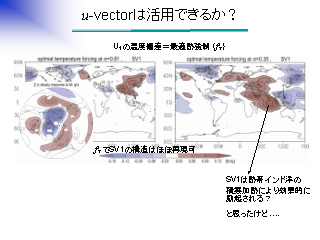
u-vector は活用できるか? (1)

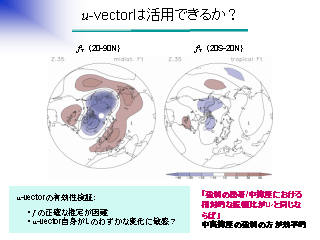
u-vector は活用できるか? (2)

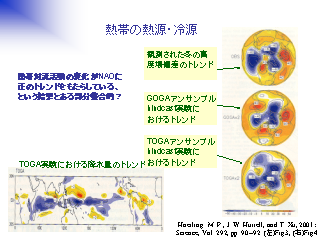
熱帯の熱源・冷源

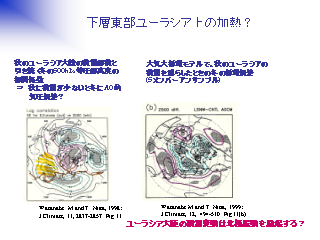
下層東部ユーラシア上の加熱?

まとめ

参考文献

• Ambaum M. H. P., B. J. Hoskins, and D. B. Stephenson, 2001: Arctic oscillation or north atlantic oscillation? J. Climate, 14, 3495-3507.

• Bladé I., 1996: On the relationship of barotropic singular modes to the low-freq uency variability of a general circulation model. J. Atmos. Sci., 53, 2393-2399.

• Branstator G., 1985a: Analysis of general circulation model sea-surface temperature anomaly simulationsusing a linear model. part I: Forced solutions. J. Atmos. Sci., 42, 2225-2241.

• Branstator G., 1985b: Analysis of general circulation model sea-surface temperature anomaly simulations using a linear model. part II: Eigenanalysis. J. Atmos. Sci., 42 2242-2254.

• Branstator G., 1990: Low-frequency patterns induced by stationary waves. J. Atmos. Sci., 47, 629-648.

• G. Branstator., 1995: Organization of storm track anomalies by recurring low-frequency circulation anomalies. J. Atmos. Sci., 52, 207-226.

• Deser C., 2000: On the teleconnectivity of the 'arctic oscillation'. Geophys. Res. Lett., 27, 779-782.

• DeWeaver E., and S. Nigam, 2000a: Do stationary waves drive the zonal-mean jet anomalies of the northern winter? J. Climate, 13, 2160-2176.

• DeWeaver E., and S. Nigam, 2000: Zonal-eddy dynamics of the north atlantic oscillation. J. Climate, 13, 3893-3914.

• Dymnikov V. P., 1988: Relationship of natural orthogonal components of the fields of meteorological characteristics to the eigenfunctions of dynamical operators. Izv. Atmos. Oceanic Phys., 24, 493-496.

• Goodman J., and J. Marshall, 2003: Using neutral singular vectors to study low-frequency atmospheric variability. J. Climate, in press.

• Horel J. D., and J. M. Wallace, 1981: Planetary scale atmospheric phenomena associated with the southern oscillation. Mon. Wea. Rev., 109, 813-829.

• Hoskins B. J., and D. J. Karoly, 1981: The steady linear response of a spherical atmosphere to thermal and orographicalforcing. J. Atmos. Sci., 38, 1179-1196.

• Hoskins B. J., A. J. Simmons, and D. C. Andrews, 1977: Energy dispersion in a barotropic atmosphere. Quart. J. R. Met. Soc., 103, 553-567.

• Itoh H., 2002: True versus apparent arctic oscillation. Geophys. Res. Lett., 29, p. 10.1029/2001GL013978.

• Itoh H., and M. Kimoto, 1999: Weather regimes, low-frequency oscillations, and principal patterns of variability: A perspective of extratropical low-frequency variability. J. Atmos. Sci., 56, 2684-2705.

• Karoly D. J., R. A. Plumb, and M. Ting, 1989: Examples of the horizontal propagation of quasi-stationary waves. J. Atmos. Sci., 46 2802-2811.

• Kimoto M., F. -F. Jin, M. Watanabe, and N. Yasutomi, 2001: Zonal-eddy coupling and a neutral mode theory for the arctic oscillation. Geophys. Res. Lett., 28, 737-740.

• Limpasuvan V., and D. L. Hartmann, 1999: Eddies and the annular modes of climate variability. Geophys. Res. Lett., 26, 3133-3136.

• Marshall, J., and F. Molteni, 1993: Toward a dynamical understanding of planetary-scale flow regimes. J. Atmos. Sci., 50, 1792-1818.

• Metz W., 1994: Singular modes and low-frequency atmospheric variability. J. Atmos. Sci., 51 1740-1753.

• Navarra, A., 1993: A new set of orthonormal modes for linearized meteorological problems. J. Atmos. Sci., 50, 2569-2583

• Nitta T., 1987: Convective activities in the tropical western pacific and their impact on the northern hemisphere summer circulation. J. Meteor. Soc. Japan, 65, 373-390.

• North G. R.,1984: Empirical orthogonal functions and normal modes. J. Atmos. Sci., 41, 879-887.

• Shindell D. T., R. L. Miller, G. Schmidt, and L. Pandolfo, 1999: Simulation of recent northern winter climate trends by greenhouse-gas forcing. Nature, 399, 452-455.

• Simmons A. J., J. M. Wallace, and G. Branstator, 1983: Barotropic wave propagation and instability, and atmospheric teleconnection patterns. J. Atmos. Sci., 40, 1363-1392.

• Thompson D. W. J., and J. M. Wallace, 2001: Regional climate impacts of the northern hemisphere annular mode. Science, 293, 85-89.

• Trenberth, K. E., and D. J. Shea., 1987: On the evolution of the Southern Oscillation. Mon. Wea. Rev., 115, 3078-3096.

• http://www.meteomediterraneo.com/Rubriche.asp?IDRubrica=6

• Wallace J. M, 2000: North atlantic oscillation/annular mode: Two paradigms--one phenomenon. Quart. J. R. Met. Soc., 126, 791-805.

• Wallace, J. M., and D. S. Gutzler, 1981: Teleconnections in the geopotential heightfield during the northern hemisphere winter. Mon. Wea. Rev., 109, 784-812.

• Wallace J. M., and D. W. J. Thompson, 2002: The pacific center of action of the northern hemisphere annular mode: Realor artifact? J. Climate, 15, 1987-1991.

• Watanabe, 2003: Thompson D. W. J., and J. M. Wallace., 2001: Regional climate impacts of the northern hemisphere annular mode. Science, 293, 85-89.

• Watanabe M., and M. Kimoto, 1999: Tropical-extratropical connection in the atlantic atmosphere-ocean variability. Geophys. Res. Lett., 26, 2247-2250.

• Watanabe M., and M. Kimoto, 2000: Atmosphere-ocean thermal coupling in the north atlantic: A positive feedback. Quart. J. R. Met. Soc., 126, 3343-2369.

• Watanabe M., and M. Kimoto, 2000: On the persistence of decadal sst anomalies in the north atlantic. J. Climate, 13, 3017-3028.

• Watanabe M., and M. Kimoto, 2001: Corrigendum. Quart. J. R. Met. Soc., 127, 733-734.

• Yamazaki K., and Y. Shinya, 1999: Analysis of the arctic oscillations simulated by agcm. J. Meteor. Soc. Japan, 77, 1287-1298.

• Zhang, Y., and I. M. Held, 1999: A linear stochastic model of a GCM's midlatitude storm tracks. J. Atmos. Sci., 56, 3416-3435.