|
2. Numerical Model |
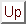


|
- Computational domain and spatial resolution of the model
(Figure 1)
-
The computational domain of the model atmosphere extends
51.2 km horizontally and 20 km vertically.
On the top of the model atmosphere,
we have added a layer where only the temperature field is
calculated to improve the accuracy of radiation flux.
Both horizontal and vertical grid intervals are 100 m except
in the lowermost 100 m height, where the vertical
levels are located at
z = 50, 25, 12.5, 6.25, and 3.125 m.
Since the staggered grid is utilized,
the lowest level at which horizontal wind is evaluated is
located at about 1.5 m height.
The value of grid interval, 100 m,
is determined by some preliminary numerical experiments
with varying the model resolution.
The vertical computational domain of the model ground surface
layer extends to six times of diurnal skin depth
δd.
The value of δd is about 8 cm (appendix A.e ).
The vertical grid interval increases with depth.
The vertical grid points normalized by
δd are located
at -0.1, -0.2, -0.35, -0.53, -0.79. -1.2, -1.8, -2.7, -4.0,
and -6.0.
- Boundary conditions
-
The horizontal boundary condition of the model atmosphere is
cyclic.
The vertical wind velocity vanishes at the surface and upper
boundary.
Above 17 km height, the numerical diffusion is introduced to
the horizontal and vertical momentum equations in order
to attenuate gravity waves excited by the thermal
convection.
The value of numerical diffusion coefficient linearly
increases from 0 to 1000
m2sec-1
between 17 and 19 km height.
The solar flux at the top of the model atmosphere
changes diurnally
under the condition of Ls = 100° at 20°N.
The seasonal condition corresponds to
the summer solstice of northern hemisphere (Ls = 90°).
The latitudinal condition is close to that of Viking Lander 1
site (22.4°N).
- Basic state and initial condition
-
The vertical temperature profile of the basic state of
model atmosphere is given as that at local time (LT) 6:00
calculated by the 1D radiative convective model that
has the same representations of radiative and ground
surface processes as those described in
Outline of the model.
The profiles of pressure and density of the basic state
are obtained from the temperature profile by the use of
the hydrostatic equation and
the equation of state for an ideal gas.
Detailed mathematical expressions of the 1D model,
calculation procedure of temperature profile, and
the actual profile adapted for the basic state
are shown in appendix C.
The initial condition for the dust-free case is a motionless
atmosphere with horizontally uniform temperature.
The vertical profile of the initial atmospheric temperature is
the same as that of the basic state mentioned above.
In order to facilitate the initial development of thermal
convection,
a random perturbation of potential temperature
with the amplitude less than 3 K
is imposed at the lowest level (z = 3.125 m).
The vertical profile of the initial ground temperature is
given as that calculated by the 1D model which is used
for determining the temperature profile of the basic
state.
The initial condition for the dusty case is described in
section 4.
- Time step and computational resources
-
The time interval of integration is 0.5 or 1 second.
Those values are determined by using CFL condition
with the phase velocity of the fastest internal gravity wave in
the model atmosphere, which is described as
where  is buoyancy frequency and is buoyancy frequency and
 is the depth of the model
atmosphere.
The time interval for integrating the radiation process
is 60 seconds,
which is a duration short enough
for radiation field to follow the temperature change
associated with the thermal convection.
This is because
the temporal scale of
temperature change associated with the thermal convection
can be estimated as 100 to 1000 sec,
provided that the magnitude of convective wind velocity
is of the order of 10 msec-1
and the depth of convection layer ranges from 1 to 10 km. is the depth of the model
atmosphere.
The time interval for integrating the radiation process
is 60 seconds,
which is a duration short enough
for radiation field to follow the temperature change
associated with the thermal convection.
This is because
the temporal scale of
temperature change associated with the thermal convection
can be estimated as 100 to 1000 sec,
provided that the magnitude of convective wind velocity
is of the order of 10 msec-1
and the depth of convection layer ranges from 1 to 10 km.
Numerical integrations were performed by using
the Fujitsu VPP 800 systems at
Kyoto University Data Processing Center and
Center for PLAnning and INformation Systems,
Institute of Space and Astronautical Science.
The size of necessary main memory was about 256M bytes.
The CPU time for executing integration of 24 model hours with
the time interval of 0.5 sec was about 8 hours.
|
A numerical simulation of thermal convection in the Martian lower
atmosphere.
Odaka, Nakajima, Ishiwatari, Hayashi,
Nagare Multimedia 2001
|


|
|